Stability Of A Second-order System
Stability of a second-order system:
As seen in the figure 1(b). The closed loop transfer function is thus
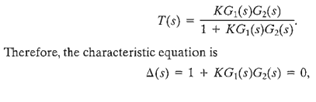
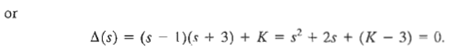
A method of obtaining the characteristic equation directly from the vector differential equation is based on the fact that the solution to the unforced system is an exponential function. The vector differential equation without input signals is

where x is the state vector. The solution is of exponential form, and we can define a constant A such that the solution of the system for one state can be of the form
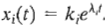
The λi are called the characteristic roots or eigenvalues of the system, which are simply the roots of the characteristic equation.If we let x = keλt and substitute into the equation above, we have

The above equation can be rewritten as:
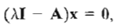
where I equals the identity matrix and 0 equals the null matrix. This set of simultaneous equations has a nontrivial solution if and only if the determinant vanishes— that is, only if
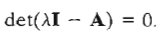
The nth-order equation in A resulting from the evaluation of this determinant is the characteristic equation, and the stability of the system can be readily ascertained.