Triple Integrals Using Cylindrical Coordinates

Triple Integrals using Cylindrical Coordinates:

Let T be a solid whose projection onto the xy-plane is labelled Ωxy. Then the solid T is the set of all points (x,y,z) satiesfying

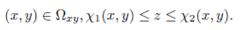
The domain Ωxy has polar coordinates in some set Ωrθ and then the solid T is cylindrical coordinates in some solid S satiesfying

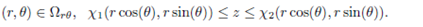
Then,

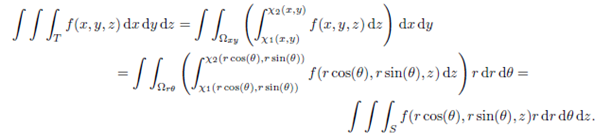
Triple Integrals Using Spherical Coordinates:

Let T be a solid xyz-space with spherical coordinates in the solid S of ρθΦ-space. Then

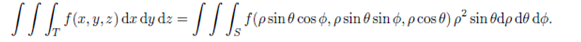
Change of Variables For Double Integrals:

Consider the change of variables x = x (u,v) and y = y (u,v) which maps the point (u,v) of some of the domain 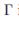into the points (x,y) of some other domain Ω. Then

Suppose now we want to integrate some function f (x,y) over Ω. If this proves difficult to do directly then we can change variables (x,y) to (u,v) and try to integrate over 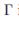instead. Then

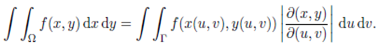
Change of Variables For Triple Integrals:

Consider the change of variables x = x (u,v,w), y = y (u,v,w), z = z (u,v,w) which maps the point (u,v,w) of some solid S into the point (x,y,z) of some other solid T. Then

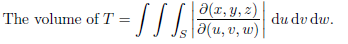
Suppose that now we want to integrate some function f (x,y,z) over T. If this proves difficult to do directly then we can change variables (x,y,z) to (u,v,w) and try to integrate over S instead. Then

Hence we can easily verify that above formula is correct for a change from Cartesian to cylindrical coordinates.