Analytical Functions
Analytical Functions:
In this chapter we consider the linear space A(Ω) of all analytic functions on an open set Ω and introduce a metric d on A(Ω) with the property that convergence in the d-metric is uniform convergence on compact subsets of Ω. We will characterize the compact subsets of the metric space (A(Ω), d) and prove several useful results on convergence of sequences of analytic functions. After these preliminaries we will present a fairly standard proof of the Riemann mapping theorem and then consider the problem of extending the mapping function to the boundary. Also included in this chapter are Runge’s theorem on rational approximations and the homotopic version of Cauchy’s theorem.
The Spaces A(Ω) and C(Ω):
Let Ω be an open subset of C. Then A ( Ω ) will denote the space of analytical functions on Ω, while C(Ω) will denote the space of all continuous functions on Ω. For n = 1,2,3....,
Let 
By basic topology of the palne, the sequence {Kn} has the following three properties:
1. Kn is compact.
2. 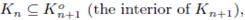
3. 
Now fix a non empty open set Ω, let {Kn} be as above and for f , g ∈ C(Ω) tehn define

Theorem:
The assignment (f,g) → d (f,g) define a metric on C(Ω). A sequence { fj } in C(Ω) is disconvergent iff { fj } is uniformly convergent on compact subset of (Ω). Thus (C(Ω), d) and (A(Ω), d) are complete metric spaces.
Proof: That d is a metric on C(Ω) is relatively straight forward. The only troublesome part of the arguments is verification of the triangle inequality, whose proof uses the inequality: If a,b,c are non negative numbers and a ≤ b c, then
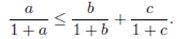
To see this notes 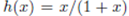 increases with x ≥ 0 and consequently h(a) ≤
increases with x ≥ 0 and consequently h(a) ≤ 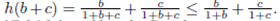 Now By using d - cauchy;
Now By using d - cauchy;
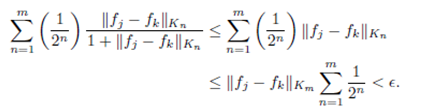
It follows that for j, k ≥ N,
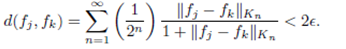
If { fn } is a sequence in A (Ω) and fn → f uniformly on compact subset of Ω, then we know that 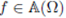 also. Hence the theroem is satiesfied.
also. Hence the theroem is satiesfied.