Rank Correlation

Rank Correlation:

When the variables under consideration are not capable of quantitative measurement but can be arranged in serial order (ranks), we find correlation between the ranks of two series. This happens when we deal with qualitative characteristics such as honesty, beauty, etc. This method is called Spearman’s Rank Difference Method or Ranking Method and the correlation coefficient so obtained is called Rank Correlation Coefficient and is denoted by rs. This method was developed by Charles  Edward Spearman, a British Psychologist in 1904. Spearman’s rank correlation coefficient is also used when the measurements are given for both the series. However, Spearman’s rank correlation coefficient rs is nothing but Karl Pearson’s correlation coefficient between the ranks, it can be interpreted in the same way as the Karl Pearson’s correlation coefficient.

Case1: When ranks are different in the two series, then

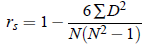
where, r_s =coefficient of rank correlation

Sum of squares of the differences in ranks (D = R_x −R_y, R_x, R_y denotes rank in x and y data.) while N = Number of Pairs

Case 2: When there is a tie i.e., if any two or more individuals have equal ranks then the formula (1) for calculating rank correlation coefficient breaks down we use the following formula:

Where m is the number of times an item is repeated. The correction factor  1/12 (m₃ − m) is to be added for each repeated value in both the series.

Computation of Rank Correlation Coefficient:

Steps (when ranks are given).

1. Compute D = R_x −R_y, the difference of ranks in the two series.

2. Compute D² and get ΣD².

3. Use the formula (8.1) or (8.2) as the case may be .

Steps (when ranks are not given).

1. Convert the given values into ranks separately for both series;

•  The highest observation is given the rank 1. The next highest observation is given rank 2 and so on. (However the rank 1 may be given to smallest observation,etc.)
• In case of a tie, we may use ‘bracket rank method’ or ‘average rank method’. In bracket rank method , we give equal ranks to the like items but the next item is given the rank as it would be in case of no ties. For example , Consider the series 20,25,22,21,22. Here 25 is given rank 1, both 22 are given rank 2
  and 2, 21 is given rank 4 (Note: Now rank 3 is not there.), 20 is given rank 5. In average rank method, common rank to be assigned to each item is the average of the ranks which these observations would have assumed if they were different. Consider the series 25,25,30,24,25,27,35. Here 35 is given rank
•  30 is given rank
• 27 is given rank 3, 25 is given rank 4 5 6
• 5 for each, 24 is given rank 7 (Note: Now rank 4 and 6 are not there).

2. Compute D, the difference of ranks.

3. Compute D² and get ΣD².

4. Use the formula (8.1) or (8.2) as the case may be .