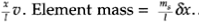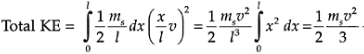Lumped Model Of A Distributed System

Lumped Model of a Distributed System

There are two broad categories of models for dynamic systems: lumped-parameter models and continuous parameter models. In a lumped-parameter model, various characteristics of the system are lumped into representative elements located at a discrete set of points in a geometric space.

 The corresponding analytical models are ordinary differential equations. Most physical systems have distributed-parameter (or continuous) components, which need spatial coordinates (e.g., x, y, z) for their representation.

These dynamic systems have time (t) and space coordinates as the independent variables. The corresponding analytical models are partial differential equations.

 For analytical convenience, we may attempt to approximate such distributed-parameter models into lumped-parameter ones. Lumped-parameter models are more commonly employed than continuous parameter models, but continuous-parameter elements sometimes are included in otherwise lumped-parameter models in order to improve the model accuracy. Let us address some pertinent issues by considering the case of a heavy spring.

A coil spring has a mass, an elastic (spring) effect, and an energy-dissipation characteristic, each of which is distributed over the entire coil.

 In an analytical model, however, these individual distributed characteristics can be approximated by a separate mass element, a spring element, and a damper element, which are interconnected in some parallel series configuration, thereby producing a lumped-parameter model. Since a heavy spring has its mass continuously distributed throughout its body, it has an infinite number of degrees of freedom.

A single coordinate cannot represent its motion. But, for many practical purposes, a lumped-parameter approximation with just one lumped mass to represent the inertial characteristics of the spring would be sufficient. Such an approximation may be obtained by using one of several approaches.

 One is the energy approach. Another approach is equivalence of natural frequency. Let us consider the energy approach first. Here we represent the spring by a lumped-parameter “model” such that the original spring and the model have the same net kinetic energy and same potential energy.

This energy equivalence is used in deriving a lumped mass parameter for the model. Even though damping (energy dissipation) is neglected in the present analysis, it is not difficult to incorporate that as well in the model.

Kinetic Energy Equivalence

Consider the uniform, heavy spring with one end fixed and the other end moving at velocity u.

Note that:

 Ms=mass of spring

 k=stiffness of spring

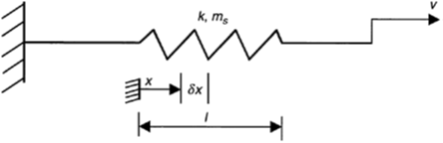
Local speed of element dx of the spring is given by