Operational Amplifier

Operational Amplifier

This is an active device (needs an external power source for operation) that can be very useful in practical circuits. With respect to the circuit element, the input output equation is

 

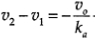
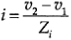
where Zi is the input impedance of the op amp. For a practical op amp Zi is very high (1 MW or more). Hence, the input current is also almost zero.

The output impedance Zo of an op amp is quite low.

 The impedance conversion property (with high Zi and low Zo) is a practical advantage of an op amp in instrumentation applications. Hence, an op amp is an impedance transformer.

Since the open-loop gain ka is quite variable and not precisely known (even though very high), an op amp is not practically used as an open-loop device.

A feedback loop is completed from the output side to an input terminal of the op amp, in order to make it stable and practically useful.

 

 

Circuit Equations

 

1. Node equations for currents: The sum of currents into a circuit node is zero. This is the well-known Kirchhoff’s current law.

2. Loop equations for voltages: The sum of voltages around a circuit loop is zero. This is the celebrated Kirchhoff’s voltage law.

 

Finally, we eliminate the unwanted (auxiliary) variables from the three types of equations (constitutive, node, loop) to obtain the analytical model (say, state equations). Linear graphs can be used for this purpose as usual.