Time-invariant Systems

Time-Invariant Systems

There is no explicit dependence on time in the functions ƒ and h, the dynamic system is said to be time-invariant, or stationary, or autonomous. In this case, the system behavior is not a function of the time origin for a given initial state and input function. In particular, a linear system is time invariant

Single-Port Elements

Single-port (or, single energy port) elements are those which can be represented by a single branch (line segment). These elements possess only one power (or energy) variable; hence the nomenclature.

Use of Linear Graphs

Linear-graph representation is particularly useful in understanding rates of energy transfer (power) associated with various phenomena, and dynamic interactions in a mechanical system can be interpreted in terms of power transfer.

 As mentioned, power is the product of a generalized force variable and the corresponding generalized velocity variable.

The total work done on a mechanical system is, in part, used as stored energy (kinetic and potential); the remainder is dissipated. Stored energy can be completely recovered when the system is brought

back to its original state (i.e., when the cycle is completed). Such a process is reversible. On the other hand, dissipation corresponds to irreversible energy transfer that cannot be recovered by returning the system to its initial state. (A fraction of the mechanical energy lost in this manner could be recovered, in principle, by operating a heat engine, but we shall not go into these details.) Energy dissipation may appear in many forms including temperature rise (a molecular phenomenon), noise (an acoustic phenomenon), or work in wear mechanisms.

The coil has a distributed mass and hence the capacity to store kinetic energy by acquiring velocity. Stored kinetic energy can be recovered as work done through a process of deceleration.

 Furthermore, the flexibility of the coil is distributed as well, and each small element in the coil has the capacity to store elastic potential energy through reversible (elastic) deflection. If the coil was moving in the vertical direction, there would be changes in gravitational potential energy, but we can disregard this in dynamic response studies if the deflections are measured from the static equilibrium position of the system. The coil will undoubtedly get warmer, make creaking noises, and wear out at the joints, clear evidence of its capacity to dissipate energy.

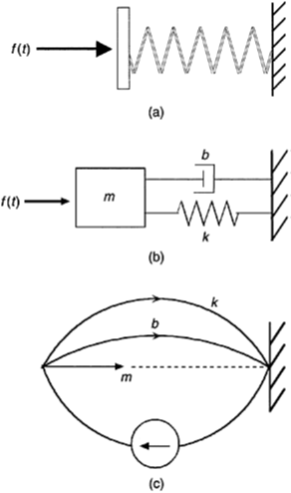
Coil spring oscillating against an external force: (a) System; (b) Lumped-parameter model; (c) Linear-graph representation of the model.