Boundry Value Problem Governed By Second Order Differential Equation

A general second order ordinary differential equation is given by
  

   y″ = f(x, y, y′), x ∈ [a, b]..................1.1

Since the ordinary differential equation is of second order, we need to prescribe two suitable conditions to obtain a unique solution of the problem. If the conditions are prescribed at the end points x = a and x = b, then it is called a two-point boundary value problem.

consider the linear second order ordinary differential equation

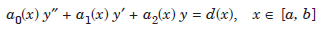....................1.2

or, in the form

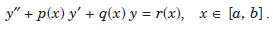...................1.3

We shall assume that the solution of Eq.(1.3) exists and is unique. This implies that a₀(x), a₁(x), a₂(x) and d(x), or p(x), q(x) and r(x) are continuous for all

x ∈ [a, b].

The two conditions required to solve Eq.(5.2) or Eq.(5.3), can be prescribed in the following three ways.

three ways:

(i) Boundary conditions of first kind The dependent variable y(x) is prescribed at the end points x = a and x = b.

y(a) = A, y(b) = B.................1.4

 

(ii) Boundary conditions of second kind The normal derivative of y(x), (slope of the solution curve) is prescribed at the end points x = a and x = b.

y′(a) = A, y′(b) = B..................1.5

 

(iii) Boundary conditions of third kind or mixed boundary conditions

 

a0 y(a) – a1 y′(a) = A,    

             
b0y(b) b1 y′(b) = B,.................1.6

 

We shall consider the solution of Eq.(5.2) or Eq.(5.3) under the boundary conditions of first kind only, that is, we shall consider the solution of the boundary value problem

y″ p(x) y′ q(x) y = r(x), x ∈ [a, b]

y(a) = A, y(b) = B..................1.7