Classification Of Linear Second Order Partial Differetiation Equation

Partial Differential Equation :Consider the general form of a second-order partial differential equation in two independent variables x and y which is linear with respect to its second-order derivatives (more precisely, we consider a semi-linear equation)

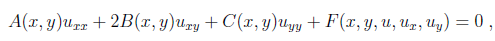................1.1

where A,B,C and F are given differentiable functions and subscripts denote partial derivatives.

The equation can be reduced to a normal form using a transformation of independent variables.

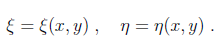...................1.2

Then Eq. (1.1) takes the form

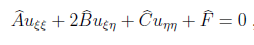
where

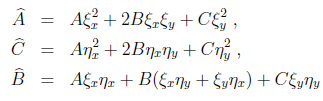
and

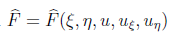
is independent of the second derivatives of u.

It can be directly verified that

so that the sign of

Definition:  Equation (1) is said to be

1.Elliptic if B2 − AC < 0 , 

2.parabolic if B2 − AC = 0 ,

3.hyperbolic if B2 − AC > 0 .

If A,B and C are functions of position, the type of a second-order PDE can be different in different regions . In three and more dimensions, second-order PDEs can be of one type in one pair of variables and of another type in the other variables, e.g., there can occur elliptic-hyperbolic equations, ultra-hyperbolic equations.