Inertial & Non-inertial Frames

Frames of References: When someone say 'the bus is moving', we would be certain that what is being described is a change of position of bus with respect to earth's surface or any building, tree etc which are fixed to the earth. We accept the local surroundings - a collection of objects attached to earth and therefore at rest relative to each other as our frame of reference. It is clear that the choice of particular frame of reference is just a matter of convenience. It is often helpful to use a frame of reference in which the description of motion is simplest. Within a frame of reference, we set up a coordinate system, which is used to manage the position of an object. To specify the position of any object, we use three numbers. The choice of number is determined by the type of coordinate system we use. The generally used coordinate systems are rectangular coordinates (x, y, z), spherical polar coordinates () and cylindrical coordinates ().

Inertial Frame and Galilean Transformation: The reference frames, which we described earlier such as earth, buildings, trees, etc., are called inertial frames. Here an object remains at rest if it is not influenced by any other forces i.e. forces arising from its interaction with other objects. Imagine a football kept on a playground, the ball remains at rest unless you kick or pick the ball (in the absence of wind). Here, the playground can be taken as an inertial frame. If one inertial frame is identified, then any other frame moving with constant velocity with respect to inertial frame is also inertial. This argument implies that all un-accelerated frames constitute the class of inertial frames.

All inertial frames are equivalent in the sense that any dynamical experiment gives same result in all these frames. Both the values of force and acceleration for an object remain same when we go from one frame to another.

Hence equation of motion F=ma gives same result in different inertial frames.   According to Galilean transformation we can show that any co-ordinate system moving uniformely with respect to an inertial system is also inertial.

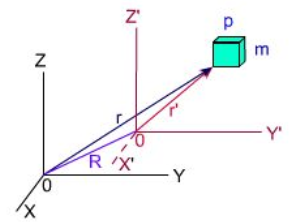
Suppose there are two observers observe the series of events such as position of body of mass m as a function of time. One is performing the experiment with respect to inertial frame and the other one is in the primed coordinate system . The primed coordinate system is in relative motion with respect to, inertial coordinate system.

Let  be the position of the mass with respect to, inertial frame and  is the position with respect to primed coordinate. The origins of two systems are displaced by R.

                              .............................................................. ( 1 )  

Taking derivatives,
                              .............................................................. ( 2 )
       and                 .............................................................. ( 3 )

If  is constant or in other words the relative motion of primed coordinate is uniform, = 0
               Or,       
                                   ...............................................................  (4 )
Where is the force due to physical interaction observed in the inertial frame and is the same force measured in the primed coordinate. The force is same in both coordinate systems. Thus the equations of motion in a system moving uniformly with respect to inertial systems are identical to those in the inertial system. All systems translating uniformly with respect to inertial systems are identical.

Of course the above arguments would be valid only if the relative motion of primed coordinate system is in no way comparable to the velocity of light. If the system moves with a velocity comparable to that of light, there would be several complications. It would be discussed later by following Einstein special theory of relativity.

               If we choose the origin of coordinate systems to coincide at t = 0, then we can write,
                        
              The time relation is generally assumed implicitly.