Intensity Distribution

Intensity distribution: We have seen in the earlier sections, that whenever two waves superimpose the resultant wave's amplitude gets modified. If the two superimposing waves are of same frequency and wave vector, then the amplitude (thus intensity) at any time and position depends upon the phase difference between the two propagating waves. As these waves are traveling waves, at a given time t, the phase of the waves vary with the position in space. Now if we consider the superposition of these waves at different points in space (at time t), the intensity will depend upon the relative phase difference between the two waves, at these points, where the phase difference between the waves in even multiple of , the intensity will be maximum and more than the sum of intensity of individual waves ; where as at points where the phase difference is odd multiple of , the intensity will be minimum and is less than the sum of intensity of indivisual waves .

At all other points, intensity will be between this maximum and minimum intensity depending upon the phase difference. Fig 1 shows the variation of intensity with phase difference between the two waves. This spatial variation of intensity gives the interference pattern on screen.

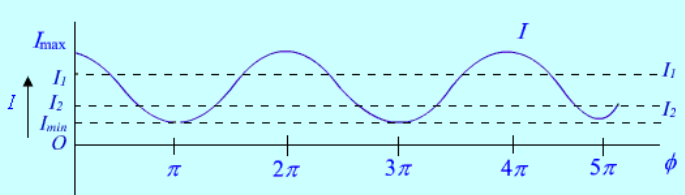fig..(1)

If the two superimposing waves are of equal amplitude then the intensity of resultant wave will vary between as the phase difference changes from . The average intensity in space however remains the same as . Thus the superposition of waves results in redistribution of energy.

Fringe visibility: In the interference pattern, the intensity of the superimposed waves is redistributed in space between I_max and I_min . The points in space, where the intensity is more, will appear brighter than the point where the intensity is less. If the intensities I_max and I_min are very different, we can clearly see the interference fringes on screen.