Torque On A Current Loop In A Uniform Magnetic Field
Torque on a Current Loop in a Uniform Magnetic Field : Though the net force on a closed current loop in a uniform magnetic field is zero, it experiences a torque. Consider a rectangular current loop PQRS of length ![]() and width
and width ![]() . The loop is in a uniform magnetic field which acts parallel to the x-axis. The loop, which is pivoted about an axis OO', carries a current
. The loop is in a uniform magnetic field which acts parallel to the x-axis. The loop, which is pivoted about an axis OO', carries a current ![]() along the direction shown in the figure.
along the direction shown in the figure.
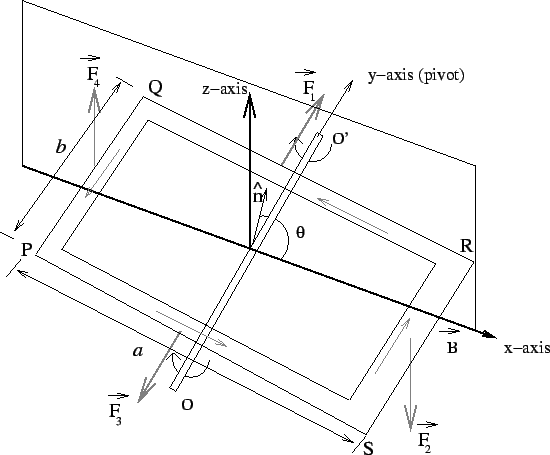
The plane of the loop (i.e. the normal ![]() the loop) makes an angle
the loop) makes an angle ![]() to the direction of the field. We take the shorter sides PQ and RS (as well as the pivot axis OO') to be perpendicular to the field direction, OO' being taken as the y-axis. The longer sides QR and QS make an angle
to the direction of the field. We take the shorter sides PQ and RS (as well as the pivot axis OO') to be perpendicular to the field direction, OO' being taken as the y-axis. The longer sides QR and QS make an angle ![]() with the field direction. Since the force on a current segment is
with the field direction. Since the force on a current segment is ![]() , the force is directed perpendicular to both
, the force is directed perpendicular to both ![]() and to the direction of the current in these segments. The force has a magnitude
and to the direction of the current in these segments. The force has a magnitude ![]() and is directed oppositely on the two sides. These forces are labelled
and is directed oppositely on the two sides. These forces are labelled ![]() and
and ![]() in the figure. (The forces are actually distributed along the lengths and the cancellation occurs for the forces acting on symmetrically placed elements on these two arms.)
in the figure. (The forces are actually distributed along the lengths and the cancellation occurs for the forces acting on symmetrically placed elements on these two arms.)
Further, since the lines of action of the forces acting on corresponding elements on these two sides are the same, there is no torque.
The forces acting on the sides PQ and RS (labelled ![]() and
and ![]() respectively) are also equal and opposite and have magnitude
respectively) are also equal and opposite and have magnitude ![]() . However, these forces do not act along the same line. The force on PQ acts parallel to
. However, these forces do not act along the same line. The force on PQ acts parallel to ![]() axis while that on RS acts parallel to
axis while that on RS acts parallel to ![]() axis. Note that
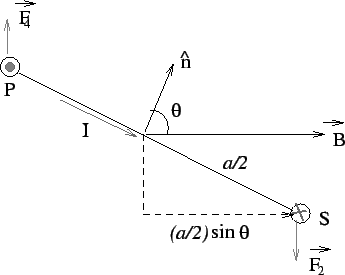
axis. Note that ![]() axis is not in the plane of the loop. The situation can be better visualized by redrawing the figure in the plane containing one of the longer sides and
axis is not in the plane of the loop. The situation can be better visualized by redrawing the figure in the plane containing one of the longer sides and ![]() .
.
The current enters the branch SR at S marked with ![]() and reenters from QP at P, marked with
and reenters from QP at P, marked with ![]() . The directions of the forces on the branches SR and QP are shown. The magnitude of the torque about the pivot is
. The directions of the forces on the branches SR and QP are shown. The magnitude of the torque about the pivot is
![]()
where ![]() is the area of the loop.
is the area of the loop.
The result above is independent of the shape of the loop. The following example for a circular loop gives an identical result.