Finite Additivity

 Introduction: Probabilistic model is the mathematical structure of any phenomenon whose input or output both are uncertain in nature they depend over some particular criteria or region.

Finite Additivity:

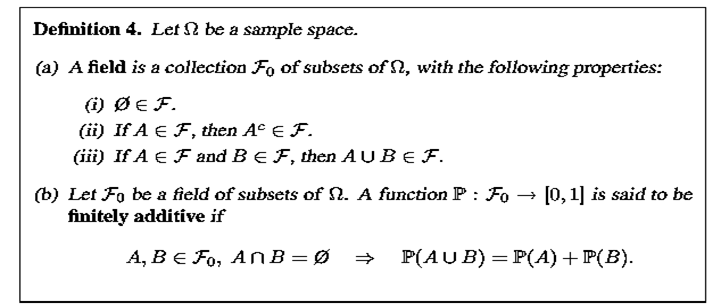
• Finite  additivity  is  strictly  weaker  than  the  countable  additivity  property  of  probability  measures.  In  particular,  finite  additivity  on  a  field,  or  even  for  the  special  case  of  a  σ-field,  does  not,  in  general,  imply  countable  additivity.
• The  reason  for  introducing  the  stronger  countable  additivity  property  is  that  with­ out  it,  we  are  severely  limited  in  the  types  of  probability  calculations  that  are  possible.  On  the  other  hand,  finite  additivity  is  often  easier  to  verify.

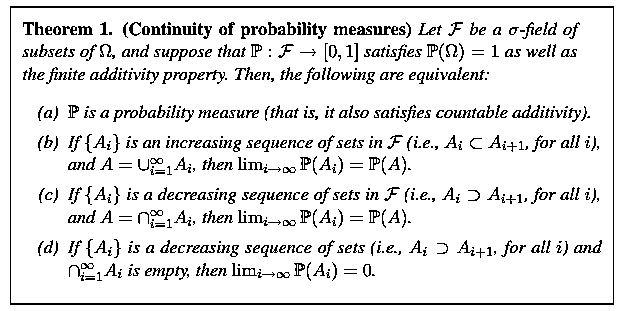
Continuity of probabilities:

• Consider a probability space in which Ω = R.  The  sequence  of  events  An = [1, n] converges  to  the  event  A = [1,∞),  and  it  is  reasonable  to  expect  that  the   probability  of  [1, n] converges  to  the  probability  of  [1,∞).
• Such  a  property  is  established  in  greater  generality  in  the  result  that  follows.  This  result  provides  us  with  a  few  alternative  versions  of  such  a  continuity  property,  together  with  a  converse  which  states  that  finite  additivity  together  with  continuity  implies  countable  additivity. 
• This  last  result  is  a  useful  tool  that  often  simplifies  the  verification  of  the  countable  additivity  property.