Origins Of The Arrays

Origins of the Arrays:

1.       It is possible that many researchers from many places in the world independently generated matrices similar to those used in standard screening using fractional factorials. Here, the focus is on the school of research started by the U.K. researcher Sir Ronald Fisher.

2.       In the associated terminology, “full factorials” are arrays of numbers that include all possible combinations of factor settings for a pre-specified number of levels.

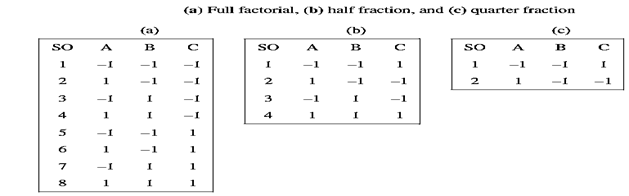
a.       For example, a full factorial with three levels and five factors consists of all 35 = 243 possible combinations. Sir Ronald Fisher generated certain fractional factorials by starting with full factorials and removing portions to create half, quarter, eighth, and other fractions.

3.       Box et al. (1961 a, b) divided fractional factorials into “regular” and “irregular” designs. “Regular fractional factorials” are experimental planning matrices that are fractions of full factorials having all of the following property.

4.       All columns in the matrix can be formed by multiplying other columns. Irregular designs are all arrays without the above-mentioned multiplicative property.

a.       For example, it can be verified that column A is equal to the product of columns B times C. Regular designs are only available with numbers of runs given by n = 2p, where p is a whole number. Therefore, possible n equal 4, 8, 16, 32… Consider the three factor full factorial and the regular fractions.

5.       The ordering of the runs in the experimental plan suggests one way to generate full factorials by alternating –1s and 1s at different rates for different columns. Note that the experimental plan is not provided in randomized order and should not be used for experimentation in the order given.

6.       The phrase “standard order” (SO) refers to the not-randomized order presented in the tables. A “generator” is a property of a specific regular fractional factorial array showing how one or more columns may be obtained by multiplying together other columns.

7.       The phrase “defining relations” refers a set of generators that are sufficiently complete as to uniquely identify a regular fractional factorial in standard order.