Time Experimentation

introduction:

 It is possible that many researchers from many places in the world independently generated matrices similar to those used in standard screening using fractional factorials. Here, the focus is on the school of research started by the U.K. researcher Sir Ronald Fisher.

Standard vs One-factor-at-a-time Experimentation:

1.       The term “standard” has been used to refer to standard screening using fractional factorials. However, other approaches are probably more “standard” or common.

2.       The phrase “one-factor-at-a-time” (OFAT) experimentation refers to the common practice of varying one factor over two levels, while holding other factors constant.

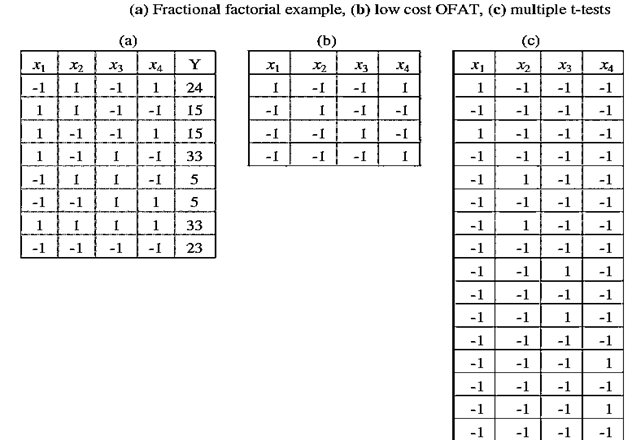
3.       After determining the importance of a single factor, focus shifts to the next factor. Table (a) shows a standard fractional factorial design and data for a hypothetical example. Table (b) and (c) show OFAT DOE plans for the same problem.

4.       The application of the plan in Table 12.13 (b) clearly has an advantage in terms of experimental costs compared with the standard method in Table 12.  runs, it would be difficult to assign any level of “proof” to the results and/or to estimate the chances of Type I or Type II errors. The design in Table (c) represents a relatively extreme attempt to achieve proof using an OFAT approach. Yet, performing two-sample t-test analyses after each set of four tests would likely result in undesirable outcomes.

5.       First, using α = 0.05 for each test, the chance of at least a single Type I error would be roughly 20%. Advanced readers can use statistical independence to estimate an error rate of 18.6%. With only n1 = n2 = 2 runs, the chances of identifying effects using this approach are even lower than the probabilities with n1 = n2 = 3. In the next, section information about Type I and II errors suggests that standard screening using fractional factorial methods offers reduced error rates of both types.