Types Of Random Variable

Independence of discrete random variables:

For  a  finite  number  of  discrete  random  variables,  independence  is  equivalent  to having a  joint  PMF  which factors  into a  product of  marginal  PMFs.

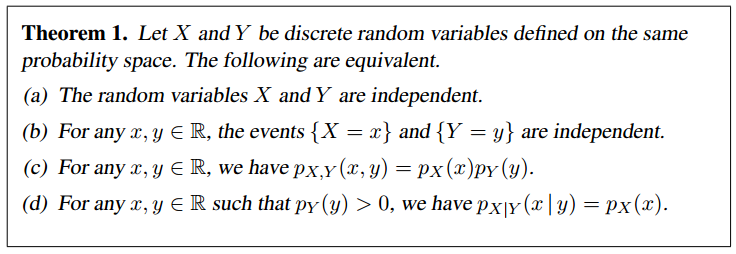
Proof:  The fact that (a) implies (b) is immediate from the definition of indepen­dence.  The  equivalence  of  (b),  (c),  and  (d)  is  also  an  immediate  consequence  of our  definitions. We complete the proof by verifying that (c) implies (a).

•       Since  this  is  true  for  any  Borel  sets  A and  B,  we  conclude  that  X and  Y are independent.
•       We note that Theorem 1 generalizes to the case of multiple, but finitely many, random variables.  The generalization of conditions (a)-(c) should be obvious. As for condition (d), it can be generalized to a few different forms, one of which is  the  following:  given  any subset  S0 of  the  random  variables  under  considera­tion,  the  conditional  joint  PMF  of  the  random  variables  Xs,  s ∈ S0,  given  the values  of  the  remaining  random  variables,  is  the  same  as  the  unconditional  joint PMF  of  the  random  variables  Xs,  s ∈ S0,  as  long  as  we  are  conditioning  on  an event  with  positive  probability.