Gain Adjustments
Gain adjustments
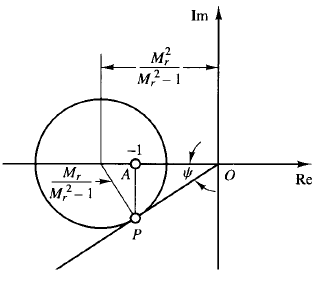
Fig 1: M-circle
The concept of M circles will now be applied to the design of control systems. In obtaining suitable performance, the adjustment of gain is usually the first consideration. The adjustment may be based on a desirable value for the resonant peak.
In the following, we shall demonstrate a method for determining the gain K so that the system will have some maximum value Mr, not exceeded over the entire frequency range.
Referring to Figure 1, we see that the tangent line drawn from the origin to the desired Mr circle has an angle of ψ, as shown, if Mr is greater than unity. The value of sin ψ is

Let us call the tangency point of the tangent line and the Mr circle as point P. It can easily be proved that the line drawn from point P, perpendicular to the negative real axis, intersects this axis at the -1 j0 point.
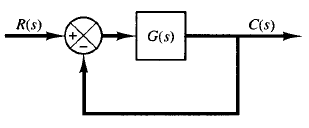
Fig 2: Control system
Consider the system shown in Figure 2. The procedure for determining the gain K so that G(jω) = KG 1(jω) will have a desired value of Mr (where Mr > 1) can be summarized as follows:
1. Draw the polar plot of the normalized open-loop transfer function G1(jω) = G(jω)/K.
2. Draw from the origin the line that makes an angle of ψ = sin-1 (1/Mr ) with the negative real axis.
3. Fit a circle with center on the negative real axis tangent to both the G1(jω) locus and the line PO.
4. Draw a perpendicular line to the negative real axis from point P, the point of tangency of this circle with the line PO. The perpendicular line PA intersects the negative real axis at point A.
5. For the circle just drawn to correspond to the desired Mr circle, point A should be the -1 j0 point.
6. The desired value of the gain K is that value which changes the scale so that point A becomes the -1 j0 point. Thus, 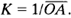
Note that the resonant frequency ωr is the frequency of the point at which the circle is tangent to the G1(jω) locus. The present procedure may not yield a satisfactory value for ωr. If this is the case, the system must be compensated in order to increase the value of ωr without changing the value of Mr.
Note also that if the system has nonunity feedback then the method requires some cut-and-try steps.