M Circles

Constant-magnitude loci (M-circles)

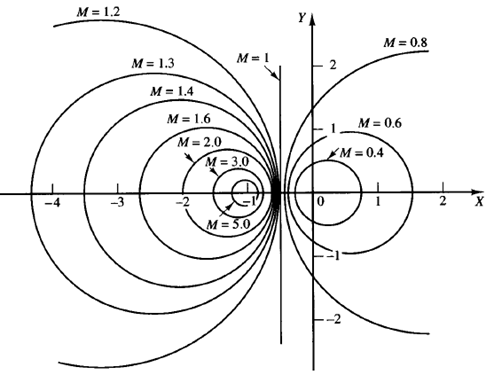
Fig: 1 A family of constant M circles

To obtain the constant-magnitude loci, let us first note that G(jω) is a complex quantity and can be written as follows:

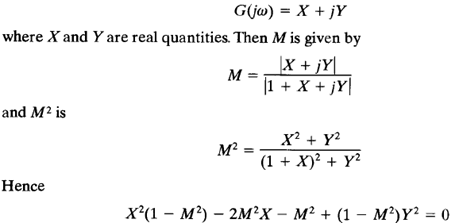
If M = 1, then from the above equation we obtain X = -1/2 This is the equation of a straight line parallel to the Y-axis and passing through the point (-t, 0).

If M ≠ 1, the above equation can be written

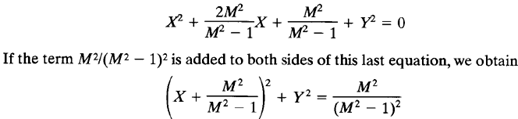
The above equation is the equation of a circle with center at X = -M²/(M² - 1), Y =0 and with radius |M/(M² - 1)|.

The constant M loci on the G(s) plane are thus a family of circles. The center and radius of the circle for a given value of M can be easily calculated.

For example, for M = 1.3, the center is at ( - 2.45,0) and the radius is 1.88.A family of constant M circles is shown in Figure 1. It is seen that as M becomes larger compared with 1, the M circles become smaller and converge to the -1 j0 point. For M > 1, the centers of the M circles lie to the left of the -1 j0 point.

Similarly, as M becomes smaller compared with 1, the M circle becomes smaller and converges to the origin. For 0 < M < 1, the centers of the M circles lie to the right of the origin. M = 1 corresponds to the locus of points equidistant from the origin and from the -1 j0 point. As stated earlier, it is a straight line passing through the point (-t, 0) and parallel to the imaginary axis. (The constant M circles corresponding to M > 1 lie to the left of the M = 1 line and those corresponding to 0< M < 1 lie to the right of the M = 1 line.) The M circles are symmetrical with respect to the straight line corresponding to M = 1 and with respect to the real axis.