Double Integral By Changing The Order Of Integration

Double Integral by Changing the Order of Integration:

In the evaluation of the double integrals sometimes we may have to change the order of integration so that evaluation is more convenient. If the limits of integration are variables then change in the order of integration changes the limits of integration. In such cases a rough idea of the region of integration is necessary.

Double Integral by Change of Variables:

Sometimes the double integrals can be evaluated easily by changing the variables. Suppose x and y are functions of two variables u and v. i.e

x = x (u,v) and y = y (u,v) and the Jacobian

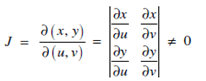
Then the region A changes into the region R under the transformations x = x (u,v) and y = y (u,v),

Then,

Examples:

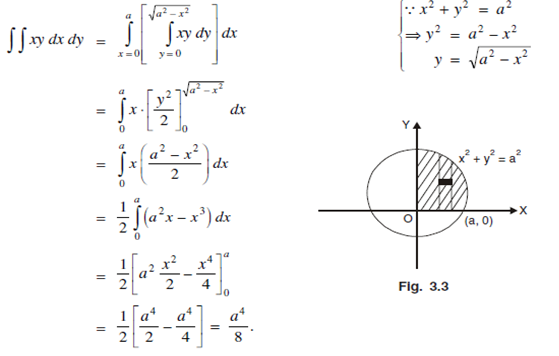
2. Evaluate 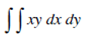over the area in the first quadranr bounded by the circle x² y² = a²

Solution: