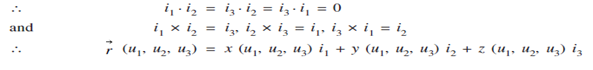Orthogonal Curvilinear Coordinates
Orthogonal Curvilinear Coordinates:
Definition: Let the coordinates (x,y,z) of any points be expressed as function of (u1,u2,u3) so that x = x (u1,u2,u3), y = y (u1,u2,u3), z = z (u1,u2,u3) then u,v,w can be expressed in terms of x,y,z as u1 = u (x,y,z), u2 = v (x,y,z), u3 = w (x,y,z). And also if 
then the system of coordinates (u1,u2,u3) will be an alternatives specification of the cartesian system (x,y,z) and (u1,u2,u3) are called the Curvilinear Coordinates of the points.
If one component is kept constant say u1 = c, then and the locus of (x,y,z) is a surface is called a coordinates surface. Thus we have three families of coordinate system corresponding to u1 = c, u2 = c, u3 = c. Suppose u1 = c, u2 = c, u3 ≠ c. in that case locus is obtained is called a coordinates curve and also there are such families.
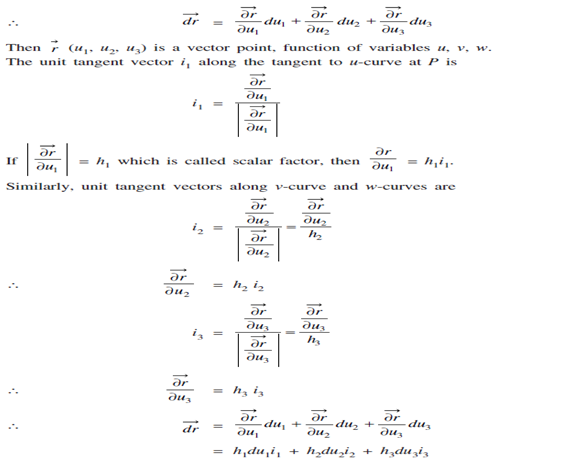
The tangent to the coordinate curves at the point P and the three coordinate axes of the curvelinear system. The direction of these axes vary from point to point and hence the unit associated with them are not constant. When the coordinates surface are manually perpendicular to each other, the three curves define an orthogonal system and (u,v,w) = (u1,u2,u3) are called Orthogonal Curvilinear Coordinates.
Unit Tangent and Unit Normal Vector:
The position vector of point P (x,y,z) is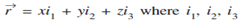 
are unit vector along the tangent to the three coordinates curves.
