Dirac Delta Generalized Function
Dirac Delta Generalized Function:
Since this is an elementary presentation of few generalized functions, we do not mention the theory of distributions, which should be the most appropriate way to present a complete theory of generalized functions. Since we restrict ourselves mainly to the Dirac delta generalized function only, we have chosen to present it as a limit of a function sequence. A continuous function sequence is a sequence whose elements are continuous functions. An example is the continuous function sequence {un}, for n ≥ 1, is given by
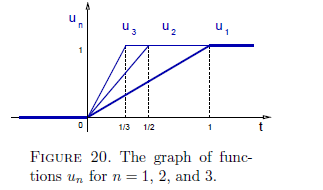
It is not difficult to see that every continuous function can be thought as the limit of a continuous function sequence. So the set of limits to continuous function sequences contains the set of all continuous functions. However, the set of limits is much bigger than the set of continuous functions. For example, it contains discontinuous functions too. The function sequence {un} is an example of a continuous function sequence whose limit is a discontinuous function. From the few graphs in Fig. 20 we can infer that lim n→ infinite un(t) = u(t), the step function, for t ≠ 0, and the limit does not exist at t = 0. So the limit is a discontinuous function, although every function in the sequence is continuous. The set of limits include even stranger objects. May be the most famous of these, which is very important to describe physical processes, is the Dirac delta generalized function. This object is the limit of certain continuous function sequences. Since there are many different sequences that have the same limit and calculations are simple with a discontinuous sequence, we introduce here the Dirac delta generalized function as the limit of a sequence of bump functions, that is, a sequence of discontinuous functions.
Theorem ( Dirac's Delta). If f : (a ,b) → R is continuous and cε (a , b), then
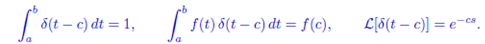
Proof of Theorem: The first equation in the above equation is simple to see from given figure and it is left as an exercise. The second equations can be proven as follows:
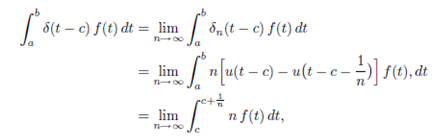
Where in the last line we used that 
If we denote by F any primitive of f, that is F' = f, then we can write,
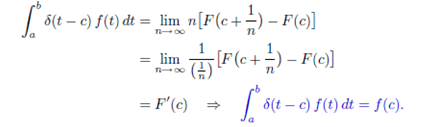
The third equation can be proven as:
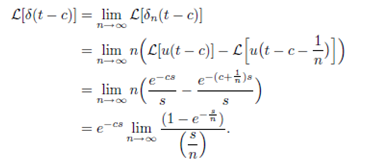
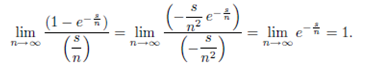
This stablish the Theroem: 