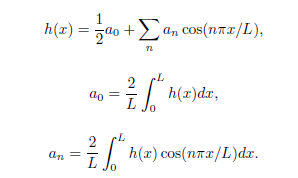Even And Odd Function
Even and Odd Function:
If f (-x) = f (x) the function, f (x) is even. This means that f (x) is symmetric about the y-axis, f (x) = x2 and f (x) = cos ( nπx/L ) are examples of even functions.
If g (-x) = - g (x) the function, g (x) is odd. This means that g (x) is anti - symmetric about the y- axis. g (x) = x3 and g (x) = sin ( nπx/L ) are examples of odd functions. A function may be neither even or odd. h (x) = exp (x) and h (x) = x cos (x) are examples of function that are neither even or odd.
Products of Function:
The product of two even function is always even. So, because f1 (x) = x2 and f2 (x) = cos (x) are both even, then h (x) = g1 (x) g2 (x) = x sinx (x) is even.
The product of two odd function is always even. So, because g1 (x) = x and g2 (x) = sin (x) are both odd, then h (x) = g1 (x) g2 (x) = x sinx (x) is even.
The product of an even function and an odd function is always odd. So, because f (x) = x2 is even and g (x) = sin (x) is odd. then h (x) = f (x) g (x) = x2 sin (x) is odd.
Integrating Even and Odd Function:
If a function is odd it is very easy to calculate its integral between limits that are symmetric about the origin,
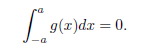
This is because 
Try drawing a graph of an odd function and comparing the area under the graph between -a and 0 to the area under the graph between 0 and a. If a function is even, then you can rewrite its integral between limits that are symmetric about the origin in the following ways,
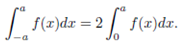
This is because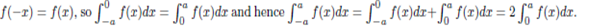 
Again you should be able to see this by examine the area under the graph of an even functions.
Sine Series: Sine Series of Fourier Series are given as:
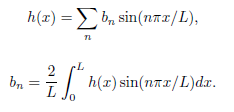
Cosine Series: Cosine Series of Fourier Series are given as:
