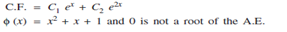Method Of Undetermined Coefficients
Method of Undetermined Coefficients:
Here we consider a method of finding the particular integral of the equation f (D) y = φ(x). When φ(x) is of some special forms. In this method first we assume that the particular integral is of certain form with some coefficients. Then substituting the value of this particular integral in the given equation and comparing the coefficients, we get the value of these “undetermined” coefficients. Therefore, the particular integral can be obtained. This method is applicable only when the equation is with constant coefficients. In the following cases we give the forms of the particular integral corresponding to a special form of φ(x).
Case(i): If φ(x) is polynomial of degree n
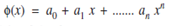
Then particular integral of the form:
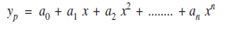
Example: φ (x) = 3x2
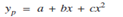
Case(ii): If φ(x) = emx then the particular integral is
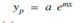
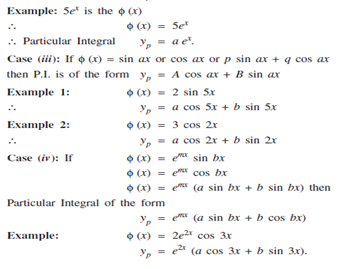
1. Solve the method of undetermined coefficients, y'' - 3y' 2y = x2 x 1.
Solution: For the given equation is ( D2 - 3D 2)y = x2 x 1
A.E is m2 - 3m 2 = 0
(m-1) (m-2) = 0
m = 1,2