Relation With Harmonic Functions

Relation with Harmonic Functions:

Analytic Functions are intimately related to harmonic functions. We say that a real-valued function h(x, y) on the plane is harmonic if it obeys Laplace's equation:

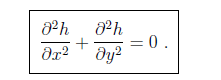
In fact, as we now show, the real and imaginary parts of an analytic function are harmonic. Let f = u i v be analytic in some open set of the complex Plane then,

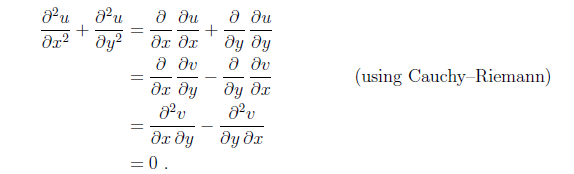
A similar calculation shows that v is also harmonic. This result is important in applications because it shows that one can obtain solutions of a second order partial differential equation by solving a system of first order partial differential equations. It is particularly important in this case because we will be able to obtain solutions of the Cauchy-Riemann equations without really solving these equations. Given a Harmonic Function u we say that another harmonic function v is its harmonic conjugate if the complex-valued function f = u i v is analytic. For example, consider the function u(x, y) = xy - x y. It is clearly harmonic since

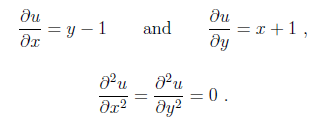
By a harmonic conjugate we mean any function v (x,y) which together with u (x,y) satiesfies the Cauchy-Riemann equations:

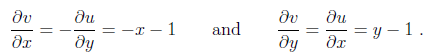
We can integrate the first of the above equations to obtain

For Ψ arbitrary functions of y which is to be determined from the second of the Cauchy-Riemann equations. Doing this one finds

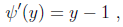
Which is solved by where C is any constant. Therefore the functions f = u iv becomes,

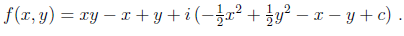
We can try to write it down in terms of z and z* by making the substitution 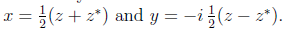After a little bit of algebra we find;

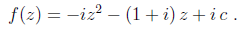
Note that all the Z* dependence has dropped out.