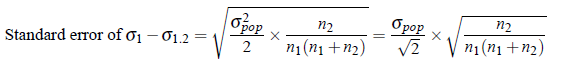Test For The Difference Of Two Sample Means
Test for the Difference of Two Sample Means:
Suppose we draw two samples from two population (or some population). Then to test the hypothesis, ‘The difference of the means of two samples is not significant or the two samples have been taken from the same population. For this we consider the difference between two sample means and the standard error of this difference. As usual, if the difference is greater than three times standard error, its considered significant otherwise not significant.
1. When population standard deviation (σpop) is known:
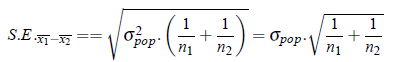
where n1 and n2 are sample sizes.
2. When population standard deviation is not known:

where σ1 is S.D. of first sample and σ2 is S.D. of second sample.
3. When a sample mean x¯1 is compared with the combined mean of two sample, (x12):
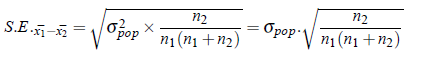
4. When the two series are correlated. That is the samples are taken from correlated populations.

Standard Error of the Difference Between two Sample Medians:
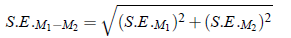
Standard Errors of Difference Between Two Sample Standard Deviations:
1. When population S.D. is known
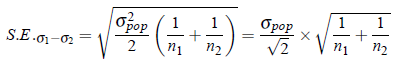
2. When population standard deviation is not known: Standard error of
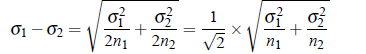
3. When standard deviation of one sample is compared with the combined standard deviation of the two samples: